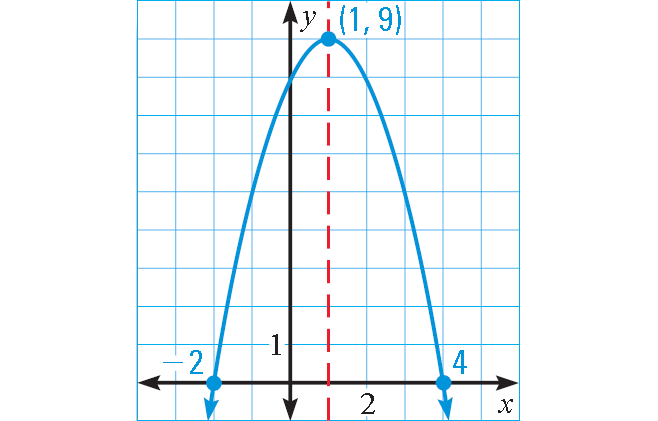
The axis of symmetry lies halfway between these points, at x = 1.
So, the x-coordinate of the vertex is x = 1 and the y-coordinate of the vertex is :
The graph of the equation is shown below.

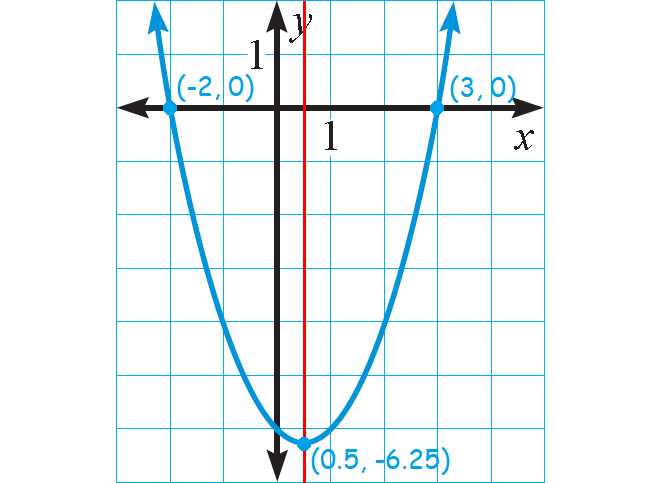
Graph : y = (x + 2)(x - 3)
The equation of the parabola is in intercept form
where a = 1, p = -2, and q = 3.
Because a > 0, the parabola opens up.
The x-intercepts occur at (-2, 0) and (3, 0).
The axis of symmetry lies halfway between these points, at x = 0.5.
So, the x-coordinate of the vertex is x = 0.5 and the y-coordinate of the vertex is :
The graph of the equation is shown below.
Graph : y = (x - 4)(x + 2)
The equation of the parabola is in intercept form
where a = 1, p = 4, and q = -2.
Because a > 0, the parabola opens up.
The x-intercepts occur at (4, 0) and (-2, 0).
Halfway of x-intercepts = 4 + (-2) / 2 ==> 1
The axis of symmetry lies halfway between these points, at x = 1.
So, the x-coordinate of the vertex is x = 1 and the y-coordinate of the vertex is :
The graph of the equation is shown below.
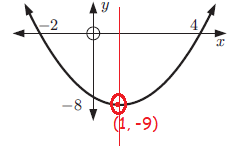
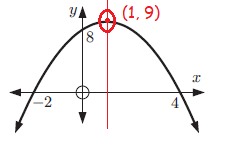
Graph : y = -(x - 4)(x + 2)
The equation of the parabola is in intercept form
where a = -1, p = 4, and q = -2.
The x-intercepts occur at (4, 0) and (-2, 0).
Halfway of x-intercepts = 4 + (-2) / 2 ==> 1
The axis of symmetry lies halfway between these points, at x = 1.
So, the x-coordinate of the vertex is x = 1 and the y-coordinate of the vertex is :
The graph of the equation is shown below.
Graph : y = 2(x + 3)(x + 5)
The equation of the parabola is in intercept form
where a = 2, p = -3, and q = -5.
Because a > 0, the parabola opens up.
The x-intercepts occur at (-3, 0) and (-5, 0).
Halfway of x-intercepts = (-3) + (-5) / 2 ==> -4
The axis of symmetry lies halfway between these points, at x = -4.
So, the x-coordinate of the vertex is x = -4 and the y-coordinate of the vertex is :
The graph of the equation is shown below.
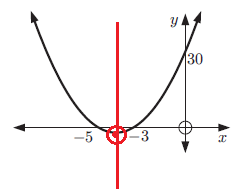
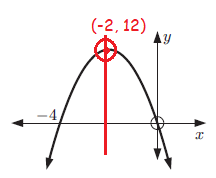
Graph : y = -3x(x + 4)
The equation of the parabola is in intercept form
where a = -3, p = 0, and q = -4.
The x-intercepts occur at (0, 0) and (-4, 0).
Halfway of x-intercepts = 0 + (-4) / 2 ==> -2
The axis of symmetry lies halfway between these points, at x = -2.
So, the x-coordinate of the vertex is x = -2 and the y-coordinate of the vertex is :
Vertex is at (-2, 12)
The graph of the equation is shown below.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
© All rights reserved. onlinemath4all.com